
MODELING
Predict and optimize yield
Background
The purpose of modeling is to carefully examine the pathways of each intended biosynthetic products, look for ways to optimize production, understand limiting factors, and to support and innovate for the team in wet lab. To accomplish these goals, we read dozens of different academic papers, sorted through metabolic pathways, and used several different methods to model acetaminophen, B12, and biomass production. Each of these modeling methods has different assumptions which allow these data points to be averaged; providing reasonable quantitative estimates of our biosynthetic products.
ACETAMINOPHEN
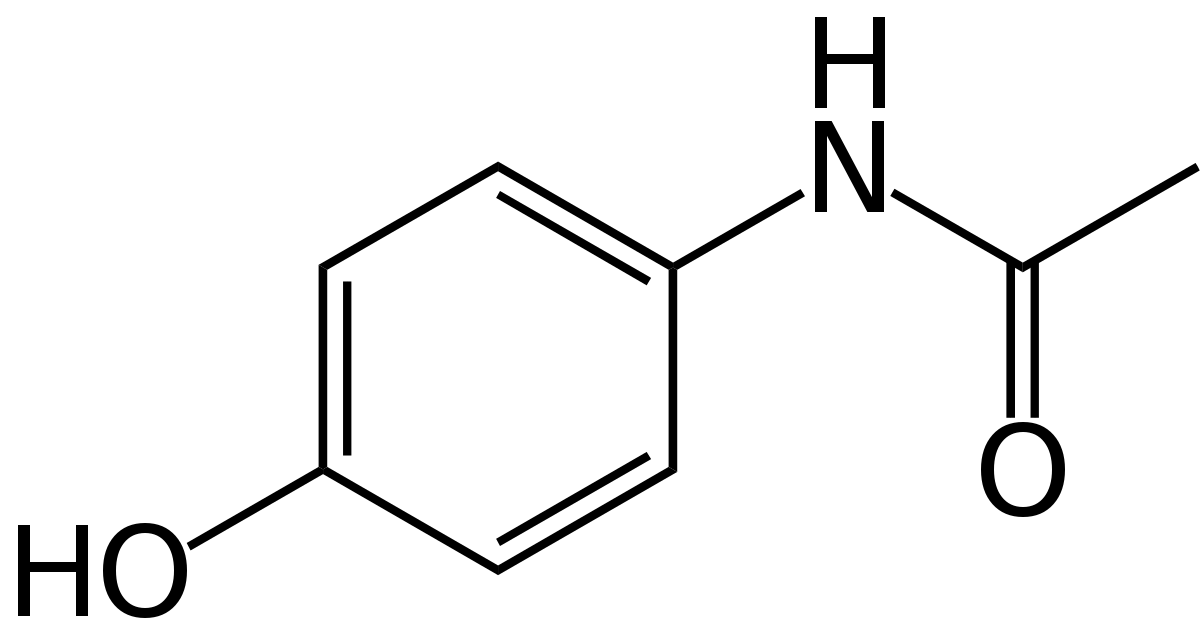
Overview
To predict acetaminophen biosynthesis, we analyzed the abundance of the acetaminophen's precursor, chorismate. Chorismate is a precursor in our organism for the three aromatic amino acids and folate[1]; producing alkaloids, salicylic acid, and vitamin K in other organisms[2]. Our gene 4ABH takes product from chorismate's tryptophan and folate pathway [3], allowing us to use published data on these products and gene transcription of tryptophan to estimate the precursor pool available to be processed into acetaminophen. Following that, we compared enzyme binding affinity Km's by creating a simulation of chorismate metabolism in Python to approximate enzyme rates and quantities. Insufficient data on cyanobacterial Km values necessitated using data from other bacterial species and comparing sequence identities to find the best match with our enzymes. With this information, we made a quantitative metabolic model which averages estimates for how much precursor goes down each pathway.

Amino Acid Method
Once we found out that acetaminophen was produced from the same precursors as the tryptophan and folate, we found published amino acid composition data for Arthrospira platensis and back converted those amino acids to moles of acetaminophen precursors anthranilate and PABA. These molecules are the direct substrate for our enzyme 4ABH, converting it to 4-aminophenol before nHoa converts it to acetaminophen. We show several different calculations below using different sources of data.
Assumptions
- Synechococcus and Arthrospira platensis have similar amino acid ratios. Since there was no available amino acid data for our transformed Synechococcus, we must assume our species has a similar ratio to the more well described Arthrospira platensis.
- The amount of tryptophan and folate are equal to the moles of their precursors, anthranilate and PABA.
- The highest matching sequence Km's would be analagous to Synechococcus's own enzyme rate.
- Of the available precursors, 30% will go down our pathway. This is based off of Km ratios of 4ABH and its competitor TrpD.
$$\frac{0.449\ mmol\ FWY}{1g\ biomass}\approx \frac{0.449\ mmol\ chor.}{1g\ biomass}\rightarrow\frac{1\ mol\ acet.}{3\ mol\ chor.}=\frac{0.15\ mmoles\ acet}{1\ g\ biomass}\times\frac{151.163g\ acet.}{1\ mol\ acet.}=\frac{22.62mg\ acet.}{1g\ biomass}$$
Sequence Analysis Method
To validate our organism's quantity of chorismate precursor, we used a custom Python program to convert DNA sequences to amino acids and calculate molar and mass percentages of chorismate derived aromatic amino acids. We ran both the genome and ribosomal protein sequences through our program, which resulted in 9.3% and 5.14% by mass aromatic amino acids. Using our higher sequence value of 9.3% and the assumption that our enzymes would take a third of the acetaminophen precursor, we estimate an acetaminophen concentration would be around 18mg per gram dried biomass.
$$\frac{0.093\ g\ FYW}{1\ g\ protein}\times\frac{0.6g protein}{1\ biomass}=\frac{0.056\ g\ FYW}{1g\ biomass}\rightarrow\frac{0.37\ mmol\ chor}{1\ g\ biomass}\times\frac{1\ mol\ acet}{3\ mol\ chor}\times\frac{151.163g\ acet}{1\ mol\ acet.}=\frac{18.61mg\ acet.}{1g\ biomass}$$$$\frac{0.051 g\ FYW}{1 g\ protein} * \frac{0.6 g\ protein}{1g\ biomass} = \frac{0.031g\ FWY}{1 g\ biomass} \rightarrow \frac{0.0.2973\ mmol\ chor}{1\ g\ biomass} * \frac{1\ mol\ acet}{3\ mol\ chor} *\frac{151.163\ g}{1 mol\ acet} = \frac{14.9\ mg\ acet}{1 g\ biomass}$$
These numbers show that there will probably be enough precursor to produce a useful, detectable quantity of acetaminophen. Based on literature and sequence estimates of aromatic amino acids, we can assume there would be at least that many moles of chorismate from which our added pathway pushes towards acetaminophen. The three calculations above can be averaged to finally predict 18.61mg ± 1.63mg acetaminophen per gram of Synechococcus biomass.
We used different chorismate concentration estimates to reach several different estimates for acetaminophen, averaging 18.61 ± 1.63mg acetaminophen per gram of Synechococcus biomass. This would be a sufficient quantity to detect through HPLC and serve as a starting point for optimizing production. This means that one 325mg dose of acetaminophen could be obtained in ~17g of biomass, meaning a 12 by 3 feet round pool could produce enough acetaminophen for more than 500 people every 10 days.
VITAMIN B12

The quantity of active form of B12 produced depends on a successful production and integration of the active B12 lower ligand, 5,6-dimethyl-benzimidazole (5,6-DMB). For phytoplankton in the wild, cobalt is often the limiting factor for growth and production of B12[5,6], while B12 production is limited by growth need in optimal media[6]. With ssuE and bluB genes inserted and regulated using a strong PrtC promoter, the activating lower ligand 5,6-DMB will be created in abundance[7]. Synechococcus and Arthrospira platensis both have CobS, bluB, and pGam genes that code for proteins which bind 5,6-DMB to the cobalt [8,9]. If these proteins work as well as in their origin organism, then assays report that 5,6-DMB has at least 100 times higher affinity for cobalt than the B12 analog ligand, adenine [10,11], meaning the DMB-B12 to B12 analog ration would be 100:1. Published HPLC results show that that Arthrospira platensis produces between 1.5-2.5µg B12 analogs per gram dry weight[12].
$$\frac{2.5µg\ B_{12}\ analog}{1g\ drymass} * \frac{100\ DMB\ bindings}{101\ DMB+adenine\ binding} = \frac{2.47µg\ active\ DMB-B_{12}}{1g\ biomass}$$An additional paper assayed Synechococcus elongatus sp WH 7803 at 10-18 moles per cell[13], and at a reported density 109 cells per liter (about a gram) [13,14], Synechococcus would produce 1.3 mµg B12 per liter dry mass. Another older paper used microbiological assays along with TLC and HPLC, finding maximums of 2.4, 1.47, and 1.27 µg B12 per gram dry mass. Averaging the 6 data points and multiplying by a 100:1 conversion ratio results in a predicted production of 1.74 ±0.23µg active DMB-B12 per gram of Arthrospira platensis drymass, meaning the USDA’s recommended daily value of 6 µg could be obtained in one 3.5 gram serving.
Assumptions
- Gene inserts will be expressed, converting riboflavin-5′-phosphate to 5,6-DMB in excess [7].
- The bluB/CobS protein complex in Arthrospira platensis will attach 5,6-DMB to cobalt at rates similar to those assayed in Propionibacterium freudenreichii[8,9].
- Cobalt will be provided in excess of 0.3 mM according the BG-11 recipe, ensuring maximum precursor availability [5,16]
- Synechococcus 7942, 7803 and Arthrospira platensis will have similar rates of B12 production.
Future B12 projects might use chemo-trophic bacteria such as Methanosarcina barkeri which produces more than 1000 times more B12 and could be converted to active form using a similar bioengineering proccess[15]. One exceptional use of cyanobacterial B12 is growing cyanobacteria in the water used to grow rice, increasing the carbon fixation, nitrogen fixation, and bioenriching the rice with B12 [12].
BIOMASS

To understand the production capacity of our organism, we aggregated growth data from published papers and all of our lab’s growth data. Using carrying-capacity-limited logistic growth curves to fit our data to an equation, we modelled dried biomass and cell count with respect to time. We have also used growth optimization papers [9,10] to add additional dependent variables of temperature, light intensity, and starter culture density to our equation.
Light Intensity: μE m-2 s-1
Temperature: ℃
Starting Density: g biomass/ L




